Theorem 1.1. If a function ![]() is defined and differentiable in the interval
is defined and differentiable in the interval ![]() and for every
and for every ![]() , then
, then ![]() is a constant in
is a constant in ![]() .
.
Application of the derivative for proving identities, inequalities and solving equations
Aim: Broadening students' knowledge about the potential of first and second derivative of a function
Application:
Proving some types of identities and inequalities as well as solving equations
Presentation structure:
Proving identities using a derivative
Theorem 1.1. If a function ![]() is defined and differentiable in the interval
is defined and differentiable in the interval ![]() and for every
and for every ![]() , then
, then ![]() is a constant in
is a constant in ![]() .
.
Corollary 1.2. If differentiable in the interval ![]() functions
functions ![]() and
and ![]() have equal derivatives everywhere in
have equal derivatives everywhere in ![]() , then they differ with a constant, i.e. if
, then they differ with a constant, i.e. if ![]() , for every
, for every ![]() , then
, then ![]() ,
, ![]() - a constant.
- a constant.
We take on trust the truthfulness of the theorem above.
Definition 1.3. Two expressions ![]() and
and ![]() (rational or irrational) are called identical in a given set
(rational or irrational) are called identical in a given set ![]() , in which
, in which ![]() and
and ![]() are defined if for all values of variables from
are defined if for all values of variables from ![]() their numerical values are equal. Then the equality
their numerical values are equal. Then the equality ![]() is called identity. Usually for
is called identity. Usually for ![]() is used the set of admissible values of the equality
is used the set of admissible values of the equality ![]() .
.
There are several ways to prove that the equality ![]() is identical with the given set
is identical with the given set ![]() :
:
Of bigger interest to us is the second method. There are cases when using traditional means (from elementary mathematics) it is hard or impossible to directly prove that ![]() . But it is possible (by means of a special technique) to show first that
. But it is possible (by means of a special technique) to show first that ![]() , and then that this
, and then that this ![]() is equal to zero.
is equal to zero.
When proving identities of the kind ![]() we implement the following algorithm:
we implement the following algorithm:
Example 1.4. Prove the identity![]() for every xq a is a constant.
for every xq a is a constant.
Solution: Let ![]() .
.
![]()
![]()
![]() . When
. When ![]() ,
, ![]()
![]() .
.
Example 1.5. Prove the identity ![]() for every x, y.
for every x, y.
Solution: Let ![]() .
.
![]()
![]()
![]() .
.
When ![]() . Consequently
. Consequently ![]() .
.
It is apparent that for most of these problems using derivatives leads to lowering of exponents of the expressions and so eases calculations.
The basic theorem can be used for proving the following identities.
Example 1.7.
![]() , if
, if ![]()
This problem represents a conditional identity which is a corollary of Example 1.6. In this case we offer a different solution.
Solution: From ![]() . Considering the function
. Considering the function
|
|
|
|
Consequently ![]() . When
. When ![]() if
if ![]() .
.
Example 1.8. Prove the identity ![]() for every a, b, c.
for every a, b, c.
Solution: Let ![]()
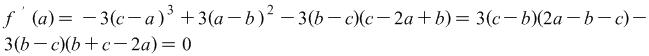
Consequently ![]() . When
. When ![]() .
.
Example 1.9.
Prove the identity
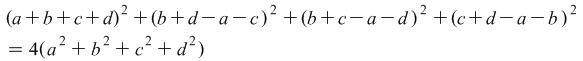
Hint: Let the function considered be ![]() .
.
Example 1.10. Prove the identity ![]() for every a, b, c.
for every a, b, c.
Solution: ![]()
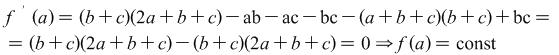
When ![]()
![]()
Example 1.11. Prove the identity![]() for every a, b, c.
for every a, b, c.
Solution: Let ![]()
![]() .
.
When ![]() .
.
Consequently ![]() .
.
Example 1.12. Prove the identity ![]() .
.
Hint:
Let ![]() .
.
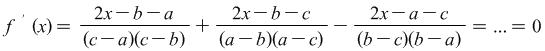
|
Consequently ![]() . When
. When ![]()
Exercise 1.13. Prove the identity ![]() for every x, y.
for every x, y.
Exercise 1.14. Prove the identity ![]() for every x,y.
for every x,y.
Exercise 1.15. Prove the identity![]() for every x, y, z.
for every x, y, z.
Exercise 1.16. Prove the identity![]() for every x, y, z.
for every x, y, z.
Exercise 1.17. Prove the identity ![]()
![]() for every a, b, x, y, z.
for every a, b, x, y, z.
Exercise 1.18. Prove the identity ![]() for every a, b, c.
for every a, b, c.
Exercise 1.19. Prove the identity ![]() for every a, b, c.
for every a, b, c.
The algorithm offered can be used for proving trigonometric identities. It has to be mentioned, though, that the derivatives of differences which result from subtraction of trigonometric expressions, rather than the identities themselves which have to be proven. This means that more complex transformations have to be carried out to prove that ![]() . This is why we will only illustrate this application with a few examples, and the rest will remain to be done individually.
. This is why we will only illustrate this application with a few examples, and the rest will remain to be done individually.
Prove the identities:
Example 2.1. Prove the identity ![]() for every x.
for every x.
Solution: Let ![]()
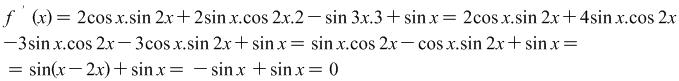
Then ![]() . When
. When ![]() the result is that
the result is that ![]()
![]() . Consequently
. Consequently ![]()
Example 2.2. Prove the identity ![]() for every x.
for every x.
Solution: Let ![]()
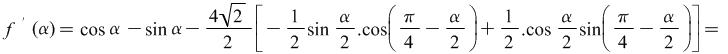
|
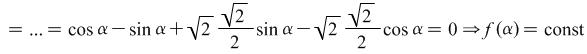
|
When ![]() .
.
Example 2.3. Prove the identity ![]() for every
for every ![]() .
.
Solution: ![]()
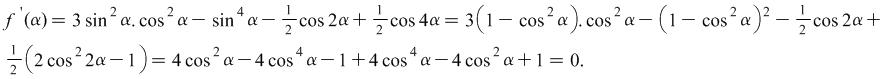
Consequently ![]() . When
. When ![]() .
.
Example 2.4. Prove the identity ![]() for every
for every ![]() .
.
Solution: Let ![]() .
.
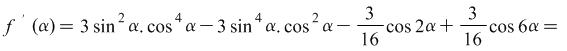
|
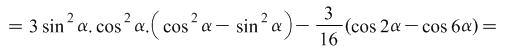
|

|
Consequently ![]() . When
. When![]()
Example 2.5. Prove the identity ![]() for every
for every ![]()
Hint:
Consider this function: ![]() . Determine that
. Determine that ![]() Establish the constant for
Establish the constant for ![]()
For trigonometric identities with two or more variables we do the following: we introduce the function ![]() , as a function of one of these variables. The remaining are treated as constants. In this case after finding the first derivative of
, as a function of one of these variables. The remaining are treated as constants. In this case after finding the first derivative of ![]() , many of the summands cease to play a part since their derivatives are zeros. And though some of the problems require a lot of calculation, they are simpler and easier.
, many of the summands cease to play a part since their derivatives are zeros. And though some of the problems require a lot of calculation, they are simpler and easier.
Example 2.6. Prove the identity ![]() for every x.
for every x.
Solution: We assume that ![]() is a constant and consider the function
is a constant and consider the function
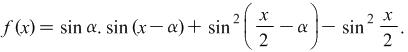
|
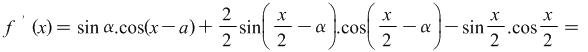
|
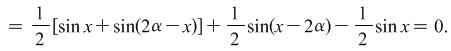
|
Consequently ![]() . Let
. Let ![]() . Then
. Then ![]() .
.
Example 2.7. Prove the identity ![]() for every
for every ![]() .
.
Solution: Let's assume that ![]() is a constant. Then
is a constant. Then
|
|
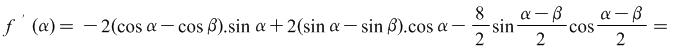
|
|
|
|
|
When ![]() , we determine that
, we determine that ![]() .
.
Example 2.8. Prove the identity ![]() for every
for every ![]()
Solution: ![]() .
.
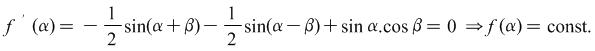
|
When ![]()
The basic theorem can be used for proving conditional trigonometric identities.
Example 2.9. Prove the identity ![]() , if
, if ![]() .
.
Solution: ![]() ,
,
|
|
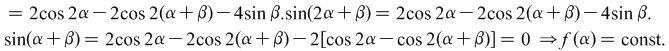
|
When ![]() .
.
Example 2.10. Prove the identity ![]() , if
, if ![]() .
.
Solution: ![]()
|
|
|
|
When![]() .
.
Example 2.11. Prove the identity ![]() if
if ![]() .
.
Solution: ![]()
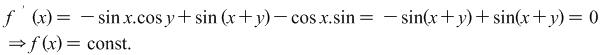
|
When ![]() . Consequently
. Consequently![]() .
.
Example 2.12. Prove the identity ![]()
![]() , if
, if ![]() ,
, ![]() .
.
Hint: Consider ![]() . Find
. Find ![]() and
and ![]() .
.
Example 2.13. Prove the identity ![]() , if
, if ![]() .
.
Hint: Let ![]()
Determine ![]() and find
and find ![]() when
when![]() .
.
Prove the following identities:
Problem 2.14.
![]() for every
for every ![]()
Problem 2.15.
![]() for every
for every ![]()
Problem 2.16.
![]()
Problem 2.17.
![]()
Problem 2.18.
![]()
Problem 2.19.
![]()
Problem 2.20.
![]()
Problem 2.21.
![]()
Problem 2.22.
![]()
Problem 2.23.
![]() , if
, if ![]()
Problem 2.24.
![]() , if
, if ![]()
Problem 2.25.
![]() , if
, if ![]()
Problem 2.26.
![]() , if
, if ![]() .
.
By Ilia Makrelov, Plovdiv university, ilmak@uni-plovdiv.bg